WORKSHEET ON BOUNDED AND UNBOUNDED INTERVALS
Problem 1 :
Convert interval notation to inequality notation or vice versa. Find the endpoints and state whether the interval is bounded, its type, and graph the interval.
(a) [-6, 3)
(b) (-∞, -1)
(c) -2 ≤ x ≤ 3
Problem 2 :
Describe and graph the interval of real numbers.
(i) x ≤ 2
(ii) -2 ≤ x < 5
Problem 3 :
Write the following as interval notation :
(i) (-∞, 7)
(ii) [-3, 3]
(iii) x is negative
(iv) x is greater than or equal to 2 and less than or equal to 6.
Problem 4 :
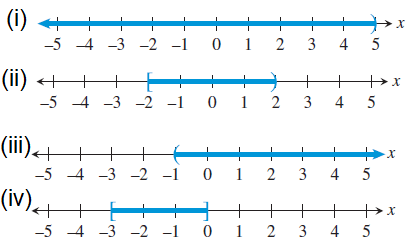
Problem 5 :
Convert to inequality notation. Find the endpoints and state whether the interval is bounded or unbounded and its type.
(i) (-3, 4]
(ii) (-3, -1)
(iii) (-∞, 5)
(iv) [-6, ∞)
Answer Key
1)
(a) It has endpoints -6 and 3.
Inequality Notation :
The possible values are -6 ≤ x < 3.
Graph :

Type of interval :
It is bounded.
(b) (-∞, -1)
Endpoints :
It has the endpoint -1.
Inequality Notation :
The possible values are x < -1.
Graph :
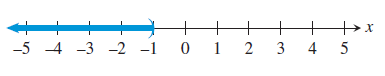
Type of interval :
It is unbounded.
(c) -2 ≤ x ≤ 3
Endpoints :
-2 and 3 are the endpoints.
Inequality Notation :
The possible values are -2 ≤ x ≤ 3.
Graph :

Type of interval :
It is bounded.
2) (i) x ≤ 2
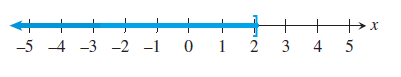
(ii) -2 ≤ x < 5
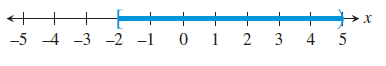
3)
(i) (-∞, 7) ==> -∞< x < 7
(ii) [-3, 3] ==> -3 ≤ x ≤ 3
(iii) x is negative ==> x < 0
(iv) x is greater than or equal to 2 and less than or equal to 6.
x ≥ 2 and x ≤ 6
4) (i) (-∞, 5) (ii) [-2, 2) (iii) (-1,∞) (iv) [-3, 0]
5) i) (-3, 4]
Inequality notation :
-3 < x ≤ 4
Endpoints :
-3 and 4 are endpoints.
Bounded : Half open
(ii) (-3, -1)
Inequality notation :
-3 < x < -1
Endpoints :
-3 and -1 are endpoints.
Bounded: Open
(iii) (-∞, 5)
Inequality notation :
x < 5
Endpoints :
End point is 5.
Unbounded : Open
(iv) [-6, ∞)
Inequality notation :
-6 < x < ∞
Endpoints :
Endpoint is -6
Unbounded : Half open
CONVERT INEQUALITIES TO INTERVAL NOTATION WORKSHEET
Write these number sets using interval notation :
Problem 1 :
{x│-1 ≤ x ≤ 6}
Problem 2 :
{x│ 0 < x < 5}
Problem 3 :
{x│-4 < x ≤ 7}
Problem 4 :
{x│ 4 ≤ x < 8}
Problem 5 :
{x│ x ≤ 2 or x ≥ 5}
Problem 6 :
{x│ x < -3 or x > 4}
Problem 7 :
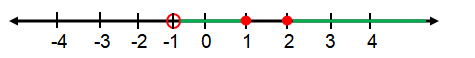
{x│ -1 < x ≤ 1 or x ≥ 2}
Problem 8 :
{x│ x < -4 or 2 ≤ x < 7}
Answer Key
1) [1, 6]

2) (0, 5).
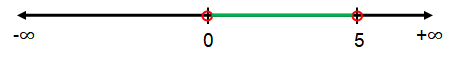
3) (-4, 7]

4) [4, 8)

5) (-∞,2] υ [5, ∞)

6) (-∞, 3) υ (4, ∞).
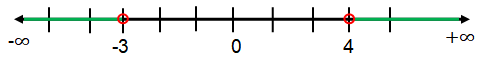
7) (-1, 1] υ [2, ∞)
8) (-∞, 4) υ [2, 7).
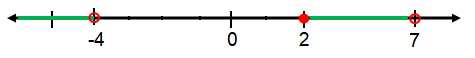
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling