FIND THE VALUES OF C THAT SATISFY THE MEAN VALUE THEOREM FOR INTEGRALS
If f is continuous on [a, b], then at some point c in [a, b],
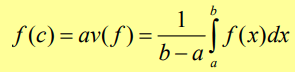
The graphical rectangular interpretation of the Mean value theorem for Definite Integrals is that:
If f is continuous on [a, b], then at some point c in [a, b] there is a rectangle with height f(c), and length b – a, such as the area of the rectangle equals the area under the curve f(x) on the interval [a, b]
For each problem, find the values of c that satisfy the Mean Value Theorem for Integrals.
Problem 1 :
f(x) = x + 2; [-3, 2]
Solution :
Finding average value of the function :
Here a = -3, b = 2
Finding the value of c that lies in the given interval :
f(x) = x + 2
f(c) = c + 2
1.5 = c + 2
c = 1.5 - 2
c = -0.5
So, the value of c is -0.5, which lies in the interval.
Problem 2 :
Solution :
Finding average value of the function :
Here a = 3, b = 5
Equating the value derived from mean value theorem for integrals to f(x), we get
So, the value of c is (9 + 2√3)/3, which lies in the interval.
Problem 3 :
Solution :
Finding average value of the function :
Here a = 2, b = 3
Equating the value derived from mean value theorem for integrals to f(x), we get
So, the value of c is √6, which lies in the interval.
Problem 4 :
Solution :
Finding average value of the function :
Here a = 0, b = 3
Equating the value derived from mean value theorem for integrals to f(x), we get
So, the value of c is 4/3, which lies in the interval.
Problem 5 :
Solution :
Finding average value of the function :
Here a = 1, b = 3
Equating the value derived from mean value theorem for integrals to f(x), we get
So, the value of c is -1 + 2√2 , which lies in the interval.
Problem 6 :
Solution :
Finding average value of the function :
Here a = 1, b = 4
Equating the value derived from mean value theorem for integrals to f(x), we get
So, the value of c is 7/3 , which lies in the interval.
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling