EVALUATE THE LIMITS FROM THE GIVEN GRAPH
Evaluating Left hand and right hand limit
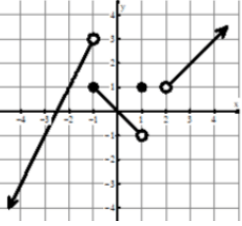
Problem :
Evaluate the following :
1) lim x->-1- f(x)
2) lim x->-1+ f(x)
Solution :
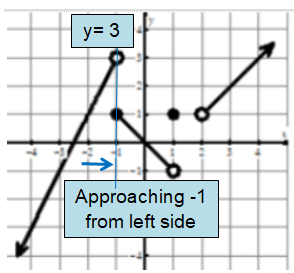
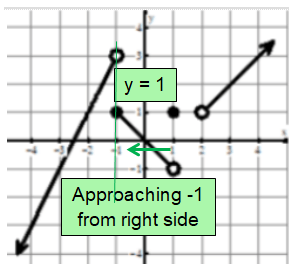
1) lim x->-1- f(x) = 3
2) lim x->-1+ f(x) = 1
When the limit does not exist ?
There are three situations
1) Jump discontinuity
2) there is a vertical asymptote
3) there is a violent oscillation
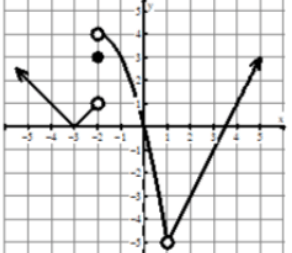
Problem :
Evaluate the following :
1) lim x->-2- f(x)
2) lim x->-2+ f(x)
3) lim x->-2 f(x)
Solution :
1) lim x->-2- f(x)
Approaching -2 from left side, we get the value of y as 1. So,
lim x->-2- f(x) = 1
2) lim x->-2+ f(x) = 4
Approaching -2 from right side, we get the value of y as 4. So,
lim x->-2+ f(x) = 4
3) lim x->-2 f(x)
Both left hand and right hand limits are not equal, the limit does not exists at x = -2.
lim x->-2 f(x) = DNE
Limit does not exists when vertical there is vertical asymptote :

lim x->2- f(x) = -inifnity
lim x->2+ f(x) = inifnity
lim x->2 f(x) = does not exists

When the limit become undefined ?
lim x->4- f(x) = 0
lim x->4+ f(x) = inifnity
lim x->4 f(x) = it is not a defined value, so undefined.
Problem 1 :
Use the graph of the function f(x) to answer each question. Use ∞, −∞ or DNE where appropriate.
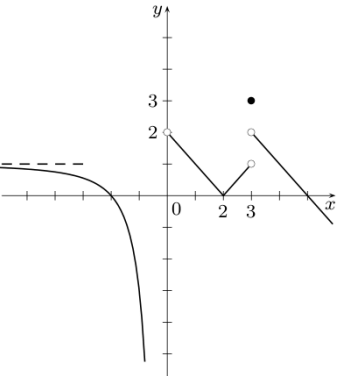
|
(a) f(0) = (b) f(2) = (c) f(3) = (d) lim x→0− f(x) |
(e) limx→0 f(x) = (f) lim x→3+ f(x) = (g) limx→3 f(x) = (h) lim x→−∞ f(x) |
Solution :
(a) f(0) :
The curve does not pass through any points on the y-axis. So, the answer is does not exists.
(b) f(2) :
The curve is passing through (2, 0). So, the value of f(2) is 0.
(c) f(3) :
We see the filled circle at (3, 3). So, the value of f(3) is 3.
(d) lim x→0− f(x) :
While approaching the value 0 from left side, the output becomes −∞. So, lim x→0− f(x) = −∞.
(e) limx→0 f(x) :
At exactly x approaches 0, the output is 2.
limx→0 f(x) = 2.
(f) lim x→3+ f(x) :
Approaching 3 from right side, the output is 2. So, lim x→3+ f(x) = 2
(g) limx→3 f(x) :
Approaching 3 from left side, we get 1. Approaching 3 from right side, we get 2. On both sides, we get different value. So, limx→3 f(x) does not exists.
(h) lim x→−∞ f(x)
At y = 1, we see the horizontal asymptote, x approaches infinity. So, the required output at x→−∞ is 1.
lim x→−∞ f(x) = 1
Problem 2 :
Use the graph of the function f(x) to answer each question. Use ∞, −∞ or DNE where appropriate.
|
(a) f(0) = (b) f(2) = (c) f(3) = |
(d) limx→−1 f(x) = (e) limx→0 f(x) = (f) lim x→2+ f(x) = (g) limx→∞ f(x) |
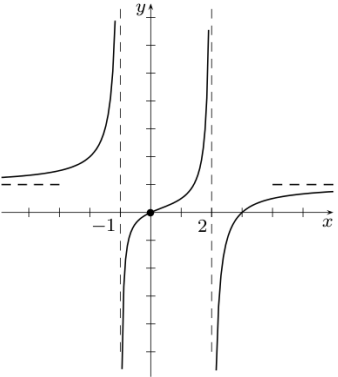
Solution :
By observing the graph above, at y = 1, we see horizontal asymptote. We see vertical asymptotes at x = -1 and x = 2,
(a) f(0) :
When the input is 0, the output also is 0. So, f(0) = 0
(b) f(2) :
At x = 2, we have vertical asymptote, we dont know where the curve is going to intersect. Then value of f(2) does not exists.
(c) f(3) :
The curve is passing through the point (3, 0). So, the value of f(3) is 0.
(d) limx→−1 f(x) :
Approaching x = -1 on either sides, from left side it approaches + infinity, from the right side it approaches - infinity. Since they are not equal, limx→−1 f(x) does not exists.
(e) limx→0 f(x) :
Approaching 0 from either sides, we see that it gives 0. So, the value of limx→0 f(x) = 0
(f) lim x→2+ f(x) :
Approaching 2 from right side, it approaches - infinity.
(g) limx→∞ f(x) :
When x approaches infinity, the output is 1. So, limx→∞ f(x) = 1
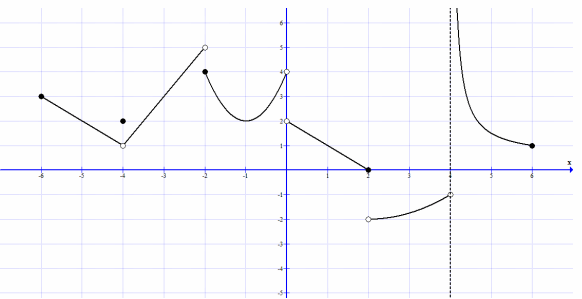
Problem 3 :

The graph of a function f is drawn above, answer the questions:
|
a) f(−4) = b) lim x→−4− f(x) = c) lim x→−4+ f(x) = d) limx→−4 f(x) = e) f(−2) = f) lim x→−2− f(x) = g) lim x→−2+ f(x) = h) limx→−2 f(x) = i) f(0) = j) lim x→0− f(x) = |
k) lim x→0+ f(x) = l) limx→0 f(x) = m) f(2) = n) lim x→2− f(x) = o) lim x→2+ f(x) = p) limx→2 f(x) = q) f(4) = r) lim x→4− f(x) = s) lim x→4+ f(x) = t) limx→4 f(x) = |
Solution :
|
a) f(−4) = 2 b) lim x→−4− f(x) = 1 c) lim x→−4+ f(x) = 1 d) limx→−4 f(x) = 1 e) f(−2) = 4 f) lim x→−2− f(x) = 5 g) lim x→−2+ f(x) = 4 h) limx→−2 f(x) = DNE i) f(0) = undefined j) lim x→0− f(x) = 4 |
k) lim x→0+ f(x) = 2 l) limx→0 f(x) = DNE m) f(2) = 0 n) lim x→2− f(x) = 0 o) lim x→2+ f(x) = -2 p) limx→2 f(x) = DNE q) f(4) = undefined r) lim x→4− f(x) = -1 s) lim x→4+ f(x) = + infinity t) limx→4 f(x) = DNE |
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling