DOMAIN RANGE AXIS OF SYMMETRY VERTEX AND ZEROES OF QUADRATIC FUNCTION
Domain :
Domain of a quadratic function is always (-∞, ∞) because quadratic function will always extent forever in either direction along the x-axis.
Range :
Range is set of all possible outputs.
Vertex :
The vertex is the turning point of the parabola.
If the parabola opens up, the vertex represents the lowest point on the graph, or the minimum value of the quadratic function.
If the parabola opens down, the vertex represents the highest point on the graph, or the maximum value.
Axis of symmetry :
The axis of symmetry is the vertical line, which divides the parabola into two equal parts.
Zeroes :
To find zeroes of the polynomial, we have to replace y by 0.
A real number k of a quadratic polynomial p(x) is 0 if
p(k) = 0
Find the important information and sketch.
(i) Vertex form
(ii) Max/Min
(iii) Axis of symmetry
(iv) Domain
(v) Range
(vi) Zeroes
(vii) y-intercept
Problem 1 :
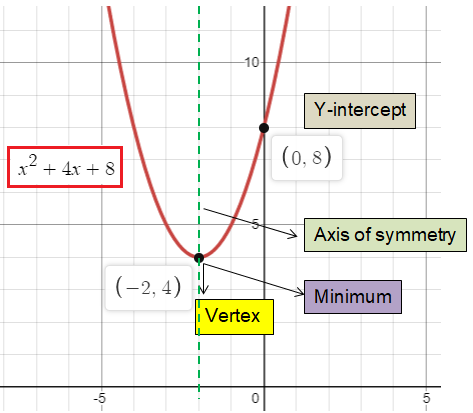
f(x) = x2 + 4x + 8
Solution :
Let f(x) = y
y = x2 + 4x + 8
(i) Vertex form :
Converting the equation into vertex for
y = a(x - h)2 + k
Writing the coefficient of x as multiple of 2.
y = x2 + 2 ⋅ x ⋅ 2 + 22 - 22 + 8
y = (x + 2)2 - 22 + 8
y = (x + 2)2 - 4 + 8
y = (x + 2)2 + 4
(h, k) ==> (-2, 4)
(ii) Maximum / Minimum :
y = 1(x + 2)2 + 4
Here a = 1 > 0, so the parabola opens up.
The given quadratic function will have minimum value.
(iii) Axis of symmetry :
x = -b/2a
f(x) = x2 + 4x + 8
Comparing the given equation with y = ax2 + bx + c
a = 1, b = 4 and c = 8
x = -4/2(1)
x = -2
(iv) Domain :
Domain of the function is -∞ < x < ∞
(v) Range :
Since the minimum value is 4, the curve will go below 4. So the range is y ≥ 4.
(vi) Zeroes :
x2 + 4x + 8 = 0
Since this quadratic function is not factorable, we will check the nature of roots.
Nature of roots = b2 - 4ac
= 42 - 4(1)(8)
= 16 - 32
= -16 < 0
So, the quadratic function has no real roots.
(vii) y-intercept :
y = x2 + 4x + 8
To find y-intercept, put x = 0
y = 02 + 4(0) + 8
y = 8
So, the y-intercept is (0, 8).
Problem 2 :
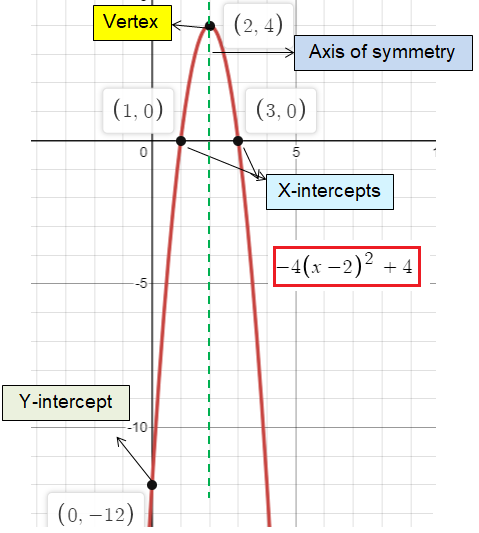
f(x) = -4(x - 2)2 + 4
Solution :
(i) Vertex form :
The given questions is in vertex form.
y = -4(x - 2)2 + 4
(h, k) ==> (2, 4)
(ii) Maximum / Minimum :
y = -4(x - 2)2 + 4
Here a = -4< 0, so the parabola opens down.
The given quadratic function will have maximum value.
(iii) Axis of symmetry :
Axis of symmetry will be at vertex.
x = -2
(iv) Domain :
Domain of the function is -∞ < x < ∞
(v) Range :
Since the minimum value is 4, the curve will go below 4. So the range is y ≥ 4.
(vi) Zeroes :
-4(x - 2)2 + 4 = 0
-4(x - 2)2 = -4
(x - 2)2 = 1
Taking square roots on both sides, we get
x - 2 = √1
x - 2 = ±1
x - 2 = 1 and x - 2 = -1
x = 1 + 2 and x = -1 + 2
x = 3 and x = 1
So, zeroes are 1 and 3.
(vii) y-intercept :
y = -4(x - 2)2 + 4
To find y-intercept, put x = 0
y = -4(0 - 2)2 + 4
y = -4(4) + 4
y = -16 + 4
y = -12
So, the y-intercept is (0, -12).
Problem 3 :
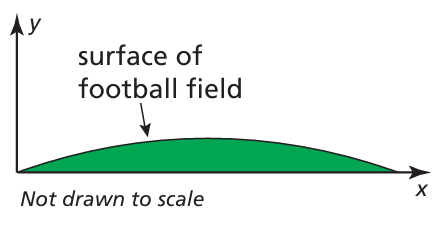
Although a football field appears to be flat, some are actually shaped like a parabola so that rain runs off to both sides. The cross section of a field can be modeled by
y = −0.000234 x (x − 160)
where x and y are measured in feet. What is the width of the field? What is the maximum height of the surface of the field?
Solution :
y = −0.000234x(x − 160)
To find the maximum height surface of the field, we find the vertex of the parabola.
y = −0.000234x2 + 160(0.000234x)
= −0.000234x2 + 0.03744x
a = -0.000234 and b = 0.03744
x = -b/2a
x = -0.03744/2(-0.000234)
= 0.03744/0.000468
x = 80
When x = 80, we get
f(80) = −0.000234(80)2 + 0.03744(80)
= -0.000234(6400) + 2.9952
= -1.4976 + 2.9952
= 1.4976
To find the width of the field, we have to find the x-intercepts.
−0.000234x(x − 160) = 0
x = 0 and x = 160
So, the width of the field is 160 ft.
So, the maximum height of the field is 1.4976 ft.
Problem 4 :
Which function represents the widest parabola? Explain your reasoning.
a) y = 2(x + 3)2 b) y = x2 − 5 c) y = 0.5(x − 1)2 + 1 d) y = −x2 + 6
Solution :
By observing value of a, we decide which parabola is widest.
Option a = 2
Option b = 1
Option c = 0.5
Option d = 1
0.5 is smallest, the option c will create a parabola which is widest.
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling