CONVERTING LOG INTO EXPONENTIAL FORM AND SOLVE

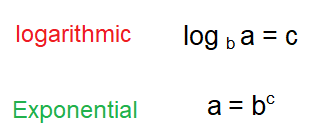
Find the value of y.

Problem 1 :

log₅ 25 = y

Solution:

y = log₅ 25

Exponential form:

5^y = 25

Writing 25 in exponential form, we get

5^y = 5²

Since bases are equal, we can equate the powers.

y = 2

Problem 2 :

log₃ 1 = y

Solution:

log₃ 1 = y

Exponential form:

3^y = 1

Anything to the power of 0 is 1.

3^y = 3⁰

Equating the powers, we get

y = 0

Problem 3 :

log₁₆ 4 = y

Solution:

log₁₆ 4 = y

y = log₁₆ 4

Exponential form:

16^y = 4

Since 16 is the multiple of 4, we can write 16 in exponential form with the base 4.

(4²)^y = 4

4^2y = 4¹

Equating the power, we get

2y = 1

y = 1/2

Problem 4 :

Solution:

Exponential form:

Problem 5 :

log₅ 1 = y

Solution:

log₅ 1 = y

Exponential form:

5^y = 1

Anything to the power of 0 is 1.

5^y = 5⁰

y = 0

Problem 6 :

log₂ 8 = y

Solution:

log₂ 8 = y

Exponential form:

2^y = 8

Writing 8 in exponential form, we get

2^y = 2³

By equating the powers, we get

y = 3

Problem 7 :

Solution:

Exponential form:

Problem 8 :

Solution:

Exponential form:

Problem 9 :

log_y 32 = 5

Solution:

log_y 32 = 5

32 = y⁵

32 can be written in exponential form.

2⁵ = y⁵

Since the powers are equal, we can equate the bases.

y = 2

Problem 10 :

Solution:

Problem 11 :

Solution:

Exponential form:

Problem 12 :

Solution:

Exponential form: