COMPOSITION OF FUNCTIONS FROM A GRAPH
Problem 1 :
Use the graph of f(x) and g(x) to find the composition of functions.
|
a. f(g(2)) b. [f ∘ g] (0) c. g(f(2)) d. [g ∘ f] (-1) |
e. g(g(4)) f. [f ∘ f] (3) g. f (g(f(1))) h. g(f(g(0))) |

Solution:
a. f(g(2))
From the graphs given above, it is clear that the line at x = 2 is crossing the curve g(x) at the point 0.
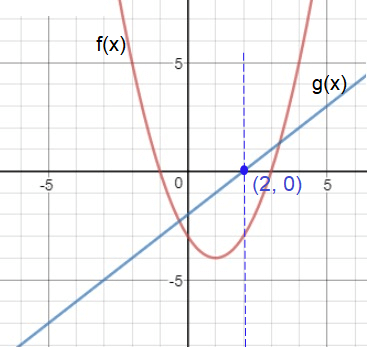
The value of g(2) is 0.
f(g(2)) = f(0)
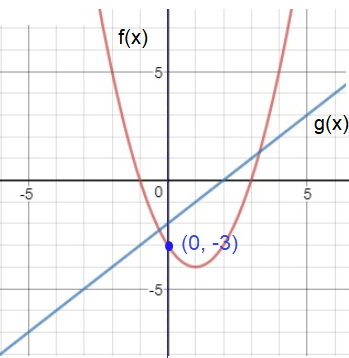
When x = 0, the vertical line crosses the curve f(x) at -3.
f(g(2)) = -3
b. [f ∘ g] (0)
From the graphs given above, it is clear that the line at x = 0 is crossing the curve g(x) at the point -2.
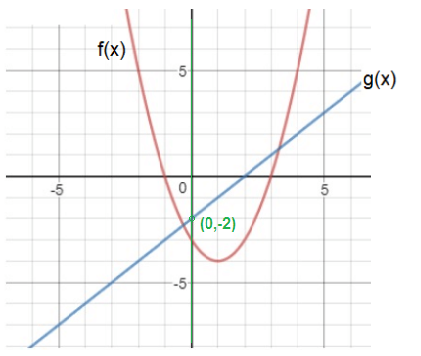
The value of g(0) is -2.
f(g(0)) = f(-2)
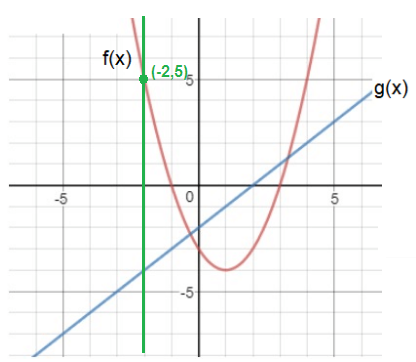
When x = -2, the vertical line crosses the curve f(x) at 5.
f(g(0)) = 5
c. g(f(2))
From the graphs given above, it is clear that the line at x = 2 is crossing the curve f(x) at the point -3.
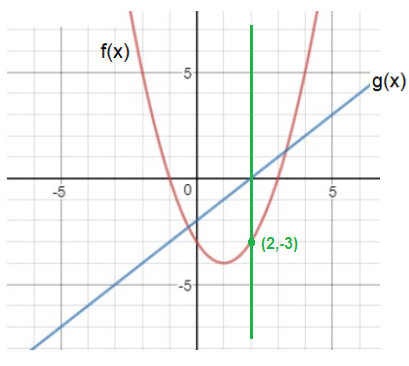
The value of f(2) is -3.
g(f(2)) = g(-3)
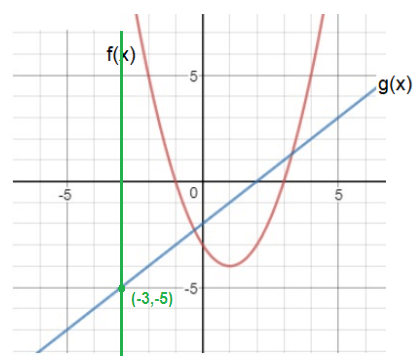
When x = -3, the vertical line crosses the curve g(x) at -5.
g(f(2)) = -5
d. [g ∘ f] (-1)
From the graphs given above, it is clear that the line is crossing the curve f(x) at 0.
The value of f(-1) is 0.
g(f(-1)) = g(0)
When x = 0, the vertical line crosses the curve g(x) at -2.
g(f(-1)) = -2
e. g(g(4))
From the graphs given above, it is clear that the line is crossing the curve g(x) at 2.
The value of g(4) is 2.
g(g(4)) = g(2)
When x = 2, the vertical line crosses the curve g(x) at 0.
g(g(4)) = 0
f. [f ∘ f] (3)
From the given graph, it is clear that the line is crossing the curve f(x) the line at 0.
The value of f(3) is 0.
f(f(3)) = f(0)
When x = 0, the vertical line crosses the curve f(x) at -3.
f(f(3)) = -3
g. f(g(f(1)))
From the given graph, it is clear that the line is crossing the curve f(x) at -4.
The value of f(1) is -4.
f(g(f(1))) = f(g(-4))
When x = -4, the vertical line crosses the curve g(x) at -6.
f(g(-4)) = -6
When x = -6, the vertical line crosses the curve f(x) at -6.
f(g(f(1))) = 45
h. g(f(g(0)))
From the given graph, it is clear that the line is crossing the curve g(x) the vertical line at -2.
The value of g(0) is -2.
g(f(g(0))) = g(f(-2))
When x = -2, the vertical line crosses the curve f(x) at 5.
g(f(-2)) = 5
When x = 5, the vertical line crosses the curve g(x) at 3.
g(f(g(0))) = 3
Problem 2 :
Use the graph to determine the value of each composite function.
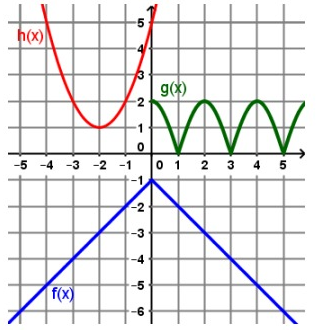
|
a. (h ∘ f) (3) = b. (f ∘ g) (4) = c. (f ∘ f) (-4) = |
d. (g ∘ g) (1) = e. (g ∘ h) (0) = |
Solution:
a. (h ∘ f) (3)
(h ∘ f) (3) = h(f(3))
From the given graph, it is clear that the line f(x) is crossing the vertical line at -4.
The value of f(3) is -4.
h(f(3)) = h(-4)
When x = -4, the vertical line crosses the curve h(x) at 5.
h(f(3)) = 5
b. (f ∘ g) (4)
(f ∘ g) (4) = f(g(4))
From the given graph, it is clear that the line is crossing the curve g(x) at 2.
The value of g(4) is 2.
f(g(4)) = f(2)
When x = 2, the vertical line crosses the curve f(x) at -3.
f(g(4)) = -3
c. (f ∘ f) (-4)
(f ∘ f) (-4) = f(f(-4))
From the given graph, it is clear that the line is crossing f(x) the curve at -5.
The value of f(-4) is -5.
f(f(-4)) = f(-5)
When x = -5, the vertical line crosses the curve f(x) at -6.
f(f(-4)) = -6
d. (g ∘ g) (1)
(g ∘ g) (1) = g(g(1))
From the given graph, it is clear that the line is crossing the curve g(x) at 0.
The value of g(1) is 0.
g(g(1)) = g(0)
When x = 0, the vertical line crosses the curve g(x) at 2.
g(g(1)) = 2
e. (g ∘ h) (0)
(g ∘ h) (0) = g(h(0))
From the given graph, it is clear that the line h(x) is crossing the vertical line at 5.
The value of h(0) is 5.
g(h(0)) = g(5)
When x = 5, the vertical line crosses the curve g(x) at 0.
g(h(0)) = 0
Problem 3 :
Use the graphs to evaluate the expressions below.
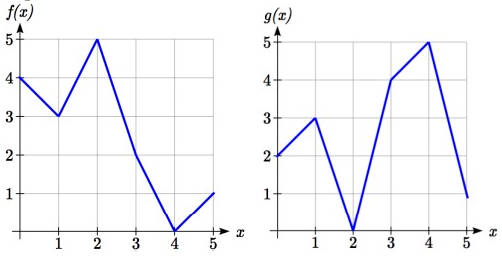
|
a. f(g(3)) b. f(g(1)) c. g(f(1)) d. g(f(0)) |
e. f(f(5)) f. f(f(4)) g. g(g(2)) h. g(g(0)) |
Solution:
a. f(g(3))
From the given graph, it is clear that the line is crossing g(x) at 4.
The value of g(3) is 4.
f(g(3)) = f(4)
When x = 4, the vertical line crosses the curve f(x) at 0.
f(g(3)) = 0
b. f(g(1))
From the given graph, it is clear that the line is crossing the g(x) at 3.
The value of g(1) is 3.
f(g(1)) = f(3)
When x = 3, the vertical line crosses the curve f(x) at 2.
f(g(1)) = 2
c. g(f(1))
From the given graph, it is clear that the line is crossing f(x) at 3.
The value of f(1) is 3.
g(f(1)) = g(3)
When x = 3, the vertical line crosses the curve g(x) at 4.
g(f(1)) = 4
d. g(f(0))
From the given graph, it is clear that the line is crossing f(x) at 4.
The value of f(0) is 4.
g(f(0)) = g(4)
When x = 4, the vertical line crosses the curve g(x) at 5.
g(f(0)) = 5
e. f(f(5))
From the given graph, it is clear that the line is crossing at f(x) at 1.
The value of f(5) is 1.
f(f(5)) = f(1)
When x = 1, the vertical line crosses the curve f(x) at 3.
f(f(5)) = 3
f. f(f(4))
From the given graph, it is clear that the line is crossing f(x) at 0.
The value of f(4) is 0.
f(f(4)) = f(0)
When x = 0, the vertical line crosses the curve f(x) at 4.
f(f(4)) = 4
g. g(g(2))
From the given graph, it is clear that the line is crossing g(x) at 0.
The value of g(2) is 0.
g(g(2)) = g(0)
When x = 0, the vertical line crosses the curve g(x) at 2.
g(g(2)) = 2
h. g(g(0))
From the given graph, it is clear that the line is crossing g(x) the vertical line at 2.
The value of g(0) is 2.
g(g(0)) = g(2)
When x = 2, the vertical line crosses the curve g(x) at 0.
g(g(0)) = 0
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling