COMPARING AVERAGE RATE OF CHANGE FOR SQUARE ROOT FUNCTION WORKSHEET

Problem 1 :

The velocity v (in meters per second) of an object in motion is given by

v(E ) = √(2E/m)

where E is the kinetic energy of the object (in joules) and m is the mass of the object (in kilograms). The mass of Object A is 4 kilograms.

The graph shows the velocity of Object B. Compare the velocities of the objects by finding and interpreting the average rates of change over the interval E = 0 to E = 6.

Solution

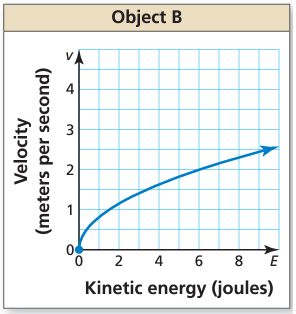
Problem 2 :

The model S(d) = √30 df represents the speed S (in miles per hour) of a van before it skids to a stop, where f is the drag factor of the road surface and d is the length (in feet) of the skid marks. The drag factor of Road Surface A is 0.75.

The graph shows the speed of the van on Road Surface B. Compare the speeds by finding and interpreting their average rates of change over the interval d = 0 to d = 15.

Solution

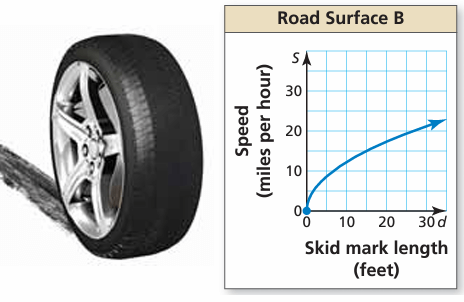
Problem 3 :

The nozzle pressure of a fire hose allows firefighters to control the amount of water they spray on a fire. The flow rate f (in gallons per minute) can be modeled by the function

f = 120√p

where p is the nozzle pressure (in pounds per square inch).

a. Use a graphing calculator to graph the function. At what pressure does the flow rate exceed 300 gallons per minute?

b. What happens to the average rate of change of the flow rate as the pressure increases?

Solution

Problem 4 :

The speed s (in meters per second) of a long jumper before jumping can be modeled by the function s = 10.9 √h , where h is the maximum height (in meters from the ground) of the jumper.

Use a graphing calculator to graph the function. A jumper is running 9.2 meters per second. Estimate the maximum height of the jumper.

Solution

Problem 5 :

The velocity v (in meters per second) of a tsunami can be modeled by the function v(x) = √(9.8x)  , where x is the water depth (in meters).

(a) Use a graphing calculator to graph the function. At what depth does the velocity of the tsunami exceed 200 meters per second?

(b) What happens to the average rate of change of the velocity as the water depth increases?

Solution