CHECKING TYPES OF DISCONTINUITY
Removable Discontinuity:
Removable discontinuities occur when a rational function has a factor with an x that exists in both the numerator and the denominator. Removable discontinuities are shown in a graph by a hollow circle that is also known as a hole. Below is the graph for
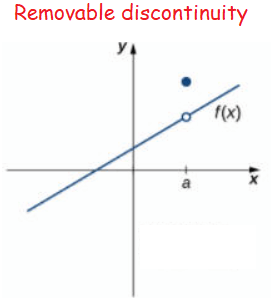
Jump discontinuity :
The function's curve would be labeled as discontinuous as it jumps from one point to another at the function's discontinuity point.
In short, the function approaches different values depending on which direction X is moving.
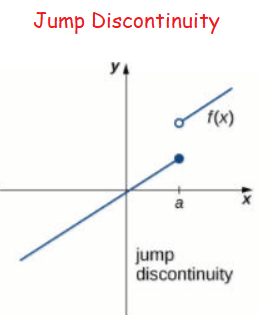
Non removable of infinite discontinuity :
An infinite discontinuity occurs when a function has a vertical asymptote on one or both sides. This is shown in the graph of the function below at x = a.
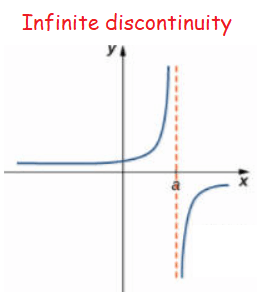
For each function identify the type of discontinuity and where it is located.
Problem 1 :
Solution :
We dont see any common factor in both numerator and denominator, so no removable discontinuity. No jump discontinuity, since there is no left hand or right hand limits.
Setting the up the denominator equal to 0 :
x + 1 = 0
x = -1
So, there is non removable discontinuity at x = -1. Vertical asymptote is at x = -1.
Problem 2 :
Solution :
Since the common factor is x, at x = 0 there is a hole. Removable discontinuity at x.
Vertical asymptote :
x + 3 = 0
x = -3
So, non removable discontinuity at x = -3.
Problem 3 :
f(x) = x3 - 4x
Solution :
For no real values of x, the functions will not become discontinuous. So, the function is continuous for all real values .
Problem 4 :
Solution :
x + 3 = 0
x = -3
So, removable discontinuity or hole at x = -3
Problem 5 :
f(x) = sec 2x for 0 ≤ x ≤ 2π
Solution :
f(x) = sec 2x
f(x) = 1/cos 2x
Vertical asymptote :
cos 2x = 0
2x = cos -1(0)
2x = π/2, 3π/2, 5π/2, 7π/2
x = π/4, 3π/4, 5π/4 and 7π/4
So, non removable discontinuities at x = π/4, 3π/4, 5π/4 and 7π/4.
Problem 6 :
Solution :
Common factor is x + 2.
x + 2 = 0
x = -2
So, removable discontinuity at x = -2.
Non removable discontinuity or hole is at x = 4.
Problem 7 :
Solution :
Common factor is x - 5.
x - 5 = 0
x = 5
So, removable discontinuity at x = 5.
Non removable discontinuity or hole is at x = 2.
Problem 8 :
Solution :
There is no common factor, to find vertical asymptote
2x - 5 = 0
2x = 5
x = 5/2
So, there is non removable discontinuity is at x = 5/2.
Problem 9 :
Solution :
The function is no undefined for any real values. So, the function is continuous for all real values.
Problem 10 :
f(x) = csc (x/2) for 0 ≤ x ≤ 2π
Solution :
f(x) = csc (x/2)
f(x) = 1/sin(x/2)
Finding the vertical asymptote,
sin (x/2) = 0
x/2 = sin-1(0)
x/2 = 0, π, 2π
x = 0, 2π
Problem 11 :
The graph of the function 𝑓(𝑥) is shown to the right: Which of the following statements is true about 𝑓?
I. 𝑓 is undefined at 𝑥 = 1.
II. 𝑓 is defined but not continuous at 𝑥 = 2.
III. 𝑓 is defined and continuous at 𝑥 = 3.
(A) Only I (B) Only II (C) I and II
(D) I and III (E) None of the statements are true.
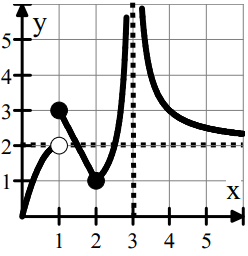
Solution :
I. 𝑓 is undefined at 𝑥 = 1.
f is defined at x = 1.
II. 𝑓 is defined but not continuous at 𝑥 = 2.
it is continuous at x = 2, because there is no hole at x = 2.
III. 𝑓 is defined and continuous at 𝑥 = 3.
Clearly, we see the vertical asymptote at x = 3.
So, none of the statement are true.
Based on the function shown in the graph give below answer the following questions.
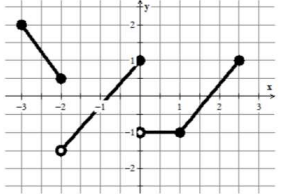
Problem 12 :
The function f(x) has removable discontinuity at
(A) x = -2 only (B) x = 0 only (C) x = 1 only
(D) x = -2 and x = 0 only
(E) f(x) has no removable discontinuities
Solution :
At x = -2 and at x = 0, the function does not exists
At x = 1, the function is continuous. To find non removable discontinuity, we should see the vertical asymptote. But we couldn't find this in the graph given above. So, option E is correct.
Problem 13 :
On what intervals is f(x) continuous ?
a) [-3, -2] U [-2, 0] U [0, 2.5] b) [-3, -2] U (-2, 0] U [0, 2.5]
c) [-3, -2] U (-2, 0] U (0, 2.5] d) [-3, -2] U [-2, 0] U (0, 2.5]
Solution :
By observing the graph, between -3 to -2, there is no discontinuity.
In between -2 to 0, there is open circle at -2 and closed circle at 0.
Between 0 to 2.5, it is continuous. So, option c is correct.
Problem 14 :
The function has jump discontinuity is at ?
Solution :
At x = -2 and at x = 0, the function has jump discontinuities.
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling