ANALYZE THE EQUATION OF A PARABOLA WORKSHEET

Write the following in standard form. Identify the 

  • Vertex
  • Focus
  • Axis of symmetry
  • Direction of opening of parabola.
  • Equation latus rectum and directrix
  • Draw the graph

Problem 1 :

y = 3x2 + 24x + 50

Solution

Problem 2 :

-6y = x2

Solution

Problem 3 :

3(y - 3) = (x - 6)2

Solution

Problem 4 :

-2(y - 4) = (x - 1)2

Solution

Problem 5 :

4(x - 2) = (y + 3)2

Solution

Answer Key

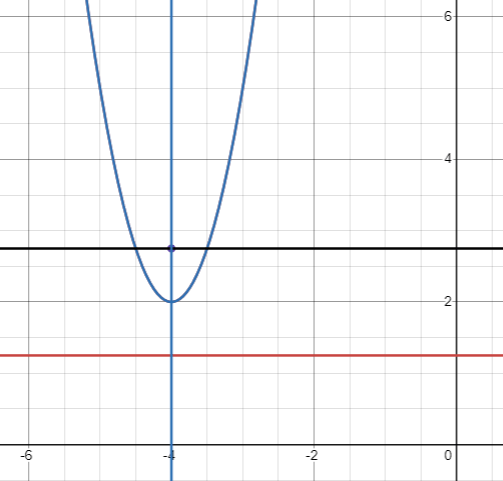
1)

Vertex

(h, k) ==> (-4, 2)

Focus

(h, k + a) 

k + a = 2 + (3/4)

= 11/4

(-4, 11/4)

Equation of latus rectum

y = k + a

y = 11/4

Equation of directrix

y = k - a

y = 2 - (3/4)

y = 5/4

Axis of symmetry

x = -4

Equation of directrix

4a = 3 units

analyze-of-parabola-q1.png

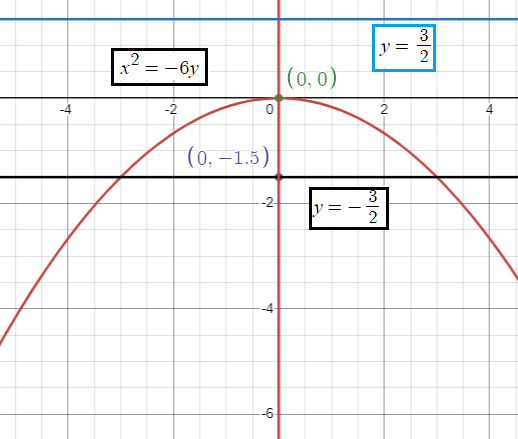
2) 

Vertex

(h, k) ==> (0, 0)

Focus

(0, -a) 

(0,-3/2)

Equation of latus rectum

y = -a

y = -3/2

Equation of directrix

y = a

y = 3/2

Axis of symmetry

x = 0

Equation of directrix

4a = 6 units

analyze-of-parabola-q2.png

3)

Vertex

(h, k) ==> (6, 3)

Focus

(h, k + a) 

k + a = 3 + (3/4)

= 15/4

(6, 15/4)

Equation of latus rectum

y = k + a

y = 15/4

Equation of directrix

y = k - a

y = 3 - (3/4)

y = 9/4

Axis of symmetry

x = 6

Equation of directrix

4a = 6 units

analyze-of-parabola-q3.png

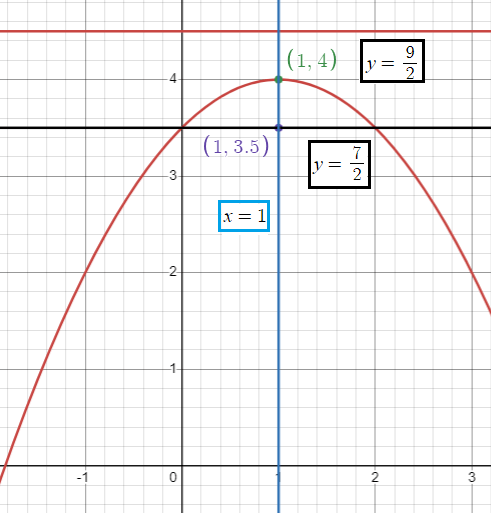
4)

Vertex

(h, k) ==> (1, 4)

Focus

(h, k - a) 

k - a = 4 - (1/2)

= 7/2

(1, 7/2)

Equation of latus rectum

y = k - a

y = 7/2

Equation of directrix

y = k + a

y = 4 + (1/2)

y = 9/2

Axis of symmetry

x = h

x = 1

Equation of directrix

4a = 2 units

analyze-of-parabola-q4.png

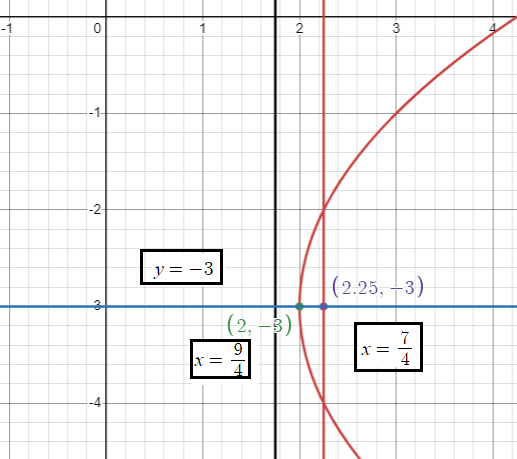
5)

Vertex

(h, k) ==> (2, -3)

Focus

(h + a, k) 

h + a = 2 + (1/4)

= 9/4

(9/4, -3)

Equation of latus rectum

x = h + a

x = 9/4

Equation of directrix

x = h - a

x = 2 - (1/4)

x = 7/4

Axis of symmetry

y = k

y = -3

Equation of directrix

4a = 1 unit

analyze-of-parabola-q5.png

Recent Articles

RSS

  1. Finding Range of Values Inequality Problems

    May 21, 24 08:51 PM

    Finding Range of Values Inequality Problems

    Read More

  2. Solving Two Step Inequality Word Problems

    May 21, 24 08:51 AM

    Solving Two Step Inequality Word Problems

    Read More

  3. Exponential Function Context and Data Modeling

    May 20, 24 10:45 PM

    Exponential Function Context and Data Modeling

    Read More