SAT PRACTICE QUESTIONS ON GEOMETRY

Problem 1 :

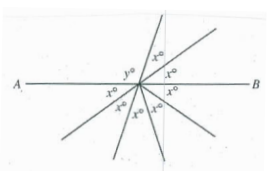
In the figure above, if AB is a line, what is the value of y?

A) 108       B) 114       C) 117    D) 120     E) 135

Solution:

By observing the figure,

2x + y = 180°

5x = 180° 

x = 180/5

x = 36

By applying x = 36 in above equation,

2(36) + y = 180

72 + y = 180

y = 180 - 72

y = 108

So, option (A) is correct.

Problem 2 :

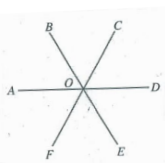
In the figure above, AD, BE and CF intersect at point O. If the measure of ∠AOB is 80° and CF bisects ∠BOD, what is the measure of ∠EOF?

A) 40°     B) 50°     C) 60°         D) 70°          E) 80°

Solution:

Given, ∠AOB = 80°

So, ∠BOD = 180 - 80 = 100°

∠BOD/2 = ∠BOC

∠BOC = 50°

∠BOC = ∠EOF (vertically opposite angles are equal)

∠EOF = 50°

So, option (B) is correct.

Problem 3 :

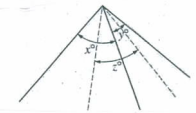
In the figure above, if x = 70 and y = 40 and the dotted lines bisect the angles with measures x° and y°, what is the value of z?

A) 30           B) 40         C) 45            D) 50            E) 55

Solution:

Given, x = 70 and y = 40

z = (x + y)/2

z = (70 + 40)/2

= 110/2

z = 55

So, option (E) is correct.

Problem 4 :

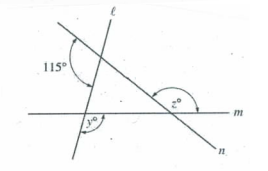
In the figure above, y + z = 

A) 180          B) 195       C) 215        D) 230      E) 245

Solution:

x + y + z = 360°

115° + y + z = 360°

y + z = 360 - 115

y + z = 245

So, option (E) is correct.

Problem 5 :

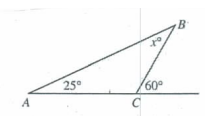
In △ABC above, what is the value of x ?

A) 25         B) 30         C) 35       D) 40       E) 60

Solution:

∠BCA = 180° - 60°

∠BCA = 120°

25 + 120 + x = 180°

145 + x = 180°

x = 35

So, option (C) is correct.

Problem 6 :

If the length of a rectangle is increased by 30% and the width of the same rectangle is decreased by 30%, what is the effect on the area of the rectangle?

A) It is increased by 60%           B) It is increased by 30%

C) It is unchanged             D) It is decreased by 15%

E) It is decreased by 9% 

Solution:

Let the length and breadth be 10 units each.

Area of rectangle = 10 × 10 = 100 sq.unit

New length = 130% of 10 = 13

New breadth = 70% of 10 = 7

So, new area of rectangle = 13 × 7 = 91 sq unit

Percentage decrease in area = (100 - 91)/100 × 100%

= 9%

So, decrease percent is 9%.

So, option (E) is correct.

Problem 7 :

What is the value of w in the figure above?

A) 90        B) 100         C) 110          D) 135       E) 145

Solution:

∠A = 180° - 35° = 145

∠B = 180° - 45° = 135

∠A + ∠B + ∠C = 180°

145 + w + 135 = 180

280 + w = 180 

w = 180 - 280

w = 100

So, option (B) is correct.

Problem 8 :

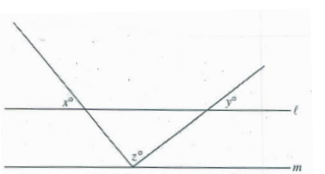
In the figure above, l||m, if x = 80 and y = 70, what is the value of z?

A) 30      B) 60       C) 75       D) 90            E) 150

Solution:

x + y + z = 180°

80 + 70 + z = 180°

150 + z = 180

z = 180 - 150

z = 30

So, option (A) is correct.

Problem 9 :

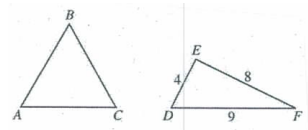
For the triangles above, the perimeter of △ABC equals the perimeter of △DEF. If △ABC is equilateral, what is the length of AB?

A) 4       B) 5         C) 7           D) 9          E) 15

Solution:

Perimeter of equilateral triangle = 4 + 8 + 9

= 21

Side of equilateral triangle = 21/3

= 7

So, length of AB is 7.

So, option (C) is correct.

Problem 10 :

A square and an equilateral triangle have equal perimeters. If the square has sides of length 3, what is the length of one side of the triangle?

A) 2       B) 3        C) 4           D) 5          E) 6

Solution:

Perimeter of square = 3 × 4

= 12

Perimeter of square = perimeter of equilateral triangle

Side of equilateral triangle = 12/3

= 4

So, option (C) is correct.